| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| 8 | 9 | 10 | 11 | 12 | 13 | 14 |
| 15 | 16 | 17 | 18 | 19 | 20 | 21 |
| 22 | 23 | 24 | 25 | 26 | 27 | 28 |
| 29 | 30 |
Tags
- string 메소드
- UI한글변경
- 구조체와 클래스의 공통점 및 차이점
- iOS14
- scanf
- correlation coefficient
- Django란
- 표준 입출력
- c++
- 연결요소
- getline
- 입출력 패턴
- 시간복잡도
- vscode
- 입/출력
- 엑셀
- string 함수
- 프레임워크와 라이브러리의 차이
- Django의 편의성
- Django Nodejs 차이점
- 알고리즘 공부방법
- 장고란
- 2557
- 백준
- double ended queue
- 자료구조
- EOF
- 매크로
- k-eta
- 이분그래프
Archives
- Today
- Total
Storage Gonie
(6) [C++, Java] 백준 No.2745 : 진법 변환 본문
반응형
문제
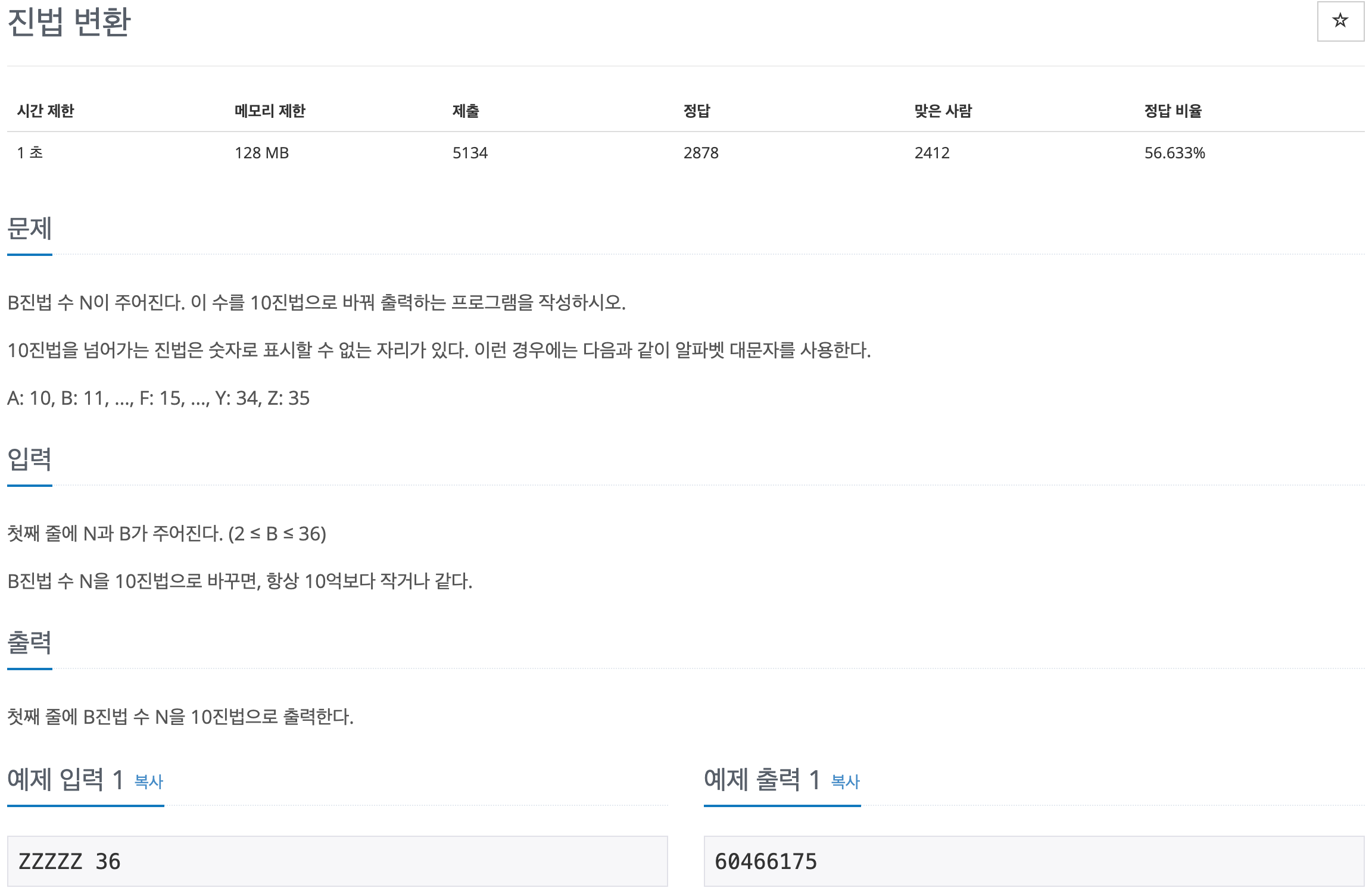
풀이
자세한 풀이 :
# C++(나의 풀이)
#include <iostream>
using namespace std;
int main() {
string N;
int B;
cin >> N >> B;
int result = 0; // 초기화 꼭해주기
for (int i = 0; i < N.size(); i++)
{
int temp;
// B진수의 각 자리값(문자)을 0 ~ 35의 숫자로 변환하기
if (N[i] <= 57) // '9' : 57
temp = N[i] - 48;
else
temp = N[i] - 55; // 'A' : 65, 'Z' : 90
// 각 자리의 수 * B^k 해주기
for(int j = 0; j < N.size()-i-1 ; j++)
temp *= B;
// 각 자리에서 계산된 값 최종 출력값에 합해주기
result += temp;
}
cout << result;
}# C++(백준 풀이)
- ans = 0;
각 자리에 대해서 ans = (ans * A) + N[i] 적용
#include <iostream>
#include <string>
using namespace std;
int main() {
int ans = 0;
string s;
int b;
cin >> s >> b;
for (int i=0; i<s.size(); i++)
{
if ('0' <= s[i] && s[i] <= '9')
ans = ans * b + (s[i] - '0');
else
ans = ans * b + (s[i] - 'A' + 10);
}
cout << ans << '\n';
}# Java
import java.util.*;
public class Main {
public static void main(String args[]) {
Scanner sc = new Scanner(System.in);
String[] line = sc.nextLine().split(" ");
String s = line[0];
int b = Integer.valueOf(line[1]);
int ans = 0;
for (int i=0; i<s.length(); i++) {
char c = s.charAt(i);
if ('0' <= c && c <= '9') {
ans = ans * b + (c - '0');
} else {
ans = ans * b + (c - 'A' + 10);
}
}
System.out.println(ans);
}
}
반응형
'알고리즘 > 백준풀이7. 수학' 카테고리의 다른 글
| (8) [C++, Java] 백준 No.1212 : 8진수 2진수 (2) | 2019.05.07 |
|---|---|
| (7) [C++, Java] 백준 No.1373 : 2진수 8진수 (0) | 2019.05.06 |
| (5) [C++, Java] 백준 No.11005 : 진법 변환2 (0) | 2019.05.04 |
| (4) [C++, Java] 백준 No.9613 : GCD 합 (0) | 2019.05.04 |
| (3) [C++, Java] 백준 No.1934 : 최소공배수 (0) | 2019.05.04 |
Comments